Technical Diving Meets Physical Chemistry

Let’s calculate how much weight our scuba cylinder will be lighter when we breathe it empty! For this we need to know one equation and a few constants from chemistry. It’s a pretty brief equation, so don’t be scared. I don’t even understand why anybody would be scared of equations anyway, but that seems to be something drilled into people these days, that “math” is hard & confusing. It’s not, in fact, it’s the opposite: it makes things clearer.

We can calculate the mass of any chunk of matter like this:
m = M n
which means that the Mass = Mass per Mol x number of Mols

A Mol is simply a very large number of molecules. The scientific community a long time ago agreed to call 6.022 x 10²³ molecules “1 Mol”. This large number, Loschmidt’s number, is simply a convention. So, to know what the mass of air in our tank is, we need to know how many Mols we have, and how much one Mol of the gas in the tank weights.

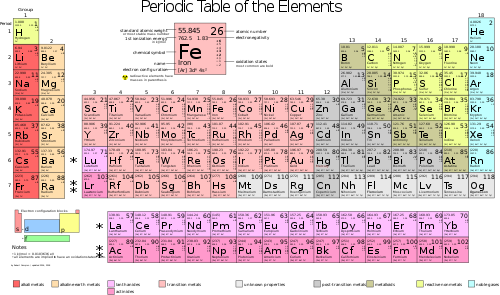
Let’s first figure out what the Mol mass of M of air is. Air is composed mainly of nitrogen and oxygen. We can look up the Mol masses (atomic masses) of oxygen and nitrogen in the periodic table of elements – these are constants of nature, specific to each element. Since both nitrogen and oxygen exist as molecules with 2 atoms, we need to multiply the number from the periodic table by two.

Oxygen: O₂ … M = 32 …. 20% of air
Nitrogen: N₂ … M = 28 ….. 80% of air
The average Mol mass, M, of air hence is 29 g/Mol
(Yes, I like to round a bit. It makes things easier, and the error we generate is small)

Now we need to know another constant of nature: there are 22.4 l per Mol at room temperature and 1 bar. A 11 l scuba tank at 200 bar obviously holds 11 x 200 liters of gas = 2200 liters. We divide this volume by the 22.4 l/Mol:

n = 2200 / 22.4 = 100 Mol of gas in our tank.
Hence we now know how many Mol we have (100) and how much a Mol of our gas weighs (29g/Mol).

n = 100
M = 29
m = n M = 100 x 29 = 2900 gram = 2.9 kgs

We find that the weight of the air in a tank filled to 200 bar is 2.9 kgs. That was easy! If we breathe the tank completely empty, we will loose 2.9 kgs of buoyancy. Since we usually leave ¼ (50 bar) of reserve in the tank during a regular dive, we will be ~ 2.2 kg lighter at the end of the dive than at the beginning.

Let’s redo the calculation for a tank full of trimix, a specialized breathing gas used for deep dives. Trimix has more Helium and less Oxygen & Nitrogen than air. A typical trimix might contain:

Oxygen: O₂ … M = 32 …. 12%
Nitrogen: N₂ … M = 28 ….. 43%
Helium He … M=4 …. 45%

average Mol mass of our trimix gas: 18g/Mol

The same 11l/200bar tank with this kind of gas will hold:

n = 100
M = 18
m = n M = 100 x 29 = 2900 gram = 1.8 kgs of gas weight.

Unsurprisingly, trimix with a lot of very light Helium is lighter than air.

Here we have it. What used to be a number we simply had to believe (Diving textbook: “You will be about 2kg lighter at the end of the dive than at the beginning”) we now understand how to calculate. Good job.